2020 Summer Game #9: Long Chain Solitaire

As I was contemplating games for this week, I tossed around a couple ideas. Not able to make up my mind, I asked Dr. Cotter which game was her favorite. Without hesitation, she said, “Long Chain Solitaire”. I told her we’d played Short Chain Solitaire earlier this year and she said this was way more fun! So, this week, we’re going to revisit our addition facts, ramp up our strategy skills, and play one of Dr. Cotter’s favorite games!

Long Chain Solitaire is from Math Card Games, game A49. It is the most challenging and enjoyable of the chain games. Like Short Chain Solitaire, we are going to build the following four columns.

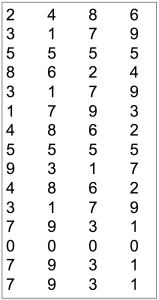
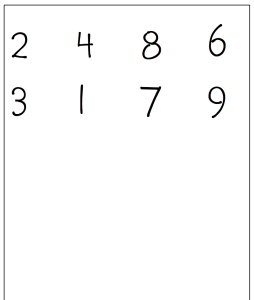
Look at the columns of numbers. See how the first two numbers in each column add up to the third number? If the two digits add up to a number over 10, the “1” from the 10 is dropped.

Let’s look at the first column. First and second numbers are 2 and 3; 2 + 3 = 5. Five is the third number. Look at the second and third numbers: 3 and 5. 3 + 5 = 8, which is the four number. Moving down, we have 5 + 8 = 13. Dropping the 1 from the 13, we have 3, which is the fifth number! See how this works?

Notice some of the patterns in the numbers? See the last four rows? Wait until the kids see this! And see how the bottom two numbers in any column add up to the top of the next column? 7 + 7 on the bottom of the first column add to up 14. There’s the 4 right at the top of the second column. 9 + 9 on the bottom of the next column is 18 and there’s the 8 at the top of the third column.

Another pattern is in the first row. Start with 2 and double it: 4. Double that and get 8. Double it again and get 16 – drop the tens and there’s the 6! Second row are numbers needed to total 5 or 15, which of course gives us a third row of all 5s. Cool, right?

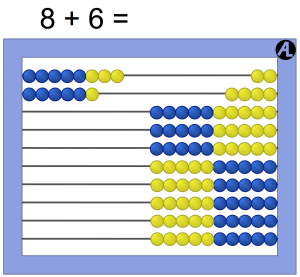
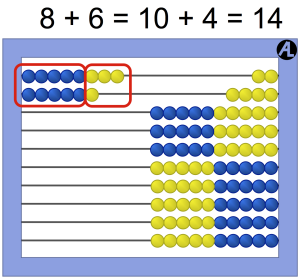
As the children are adding their way down the columns, let them use the AL Abacus to help with their addition. No, the abacus does not become a crutch. The AL Abacus is a tool that is visualizable and will become a mental image over time. So rather than finger counting to figure out what 8 plus 6 is, the child sees the abacus and, with repeated use, sees the “image” of 8 + 6 and knows the answer is 14.

Let’s get started on this week’s fun. We will need the following cards, for a total of 60 cards:

Card Number:    0    1    2    3    4    5    6    7    8    9

How many:         4    8    4    8    4    8    4    8    4    8

Neat pattern there too, with all those 4s and 8s, right? So, gather the cards, shuffle real well, then lay four cards out in a fan. Give the players the starting numbers if they are not remembering the pattern as discussed above. I’ll write them down on a piece of paper, just to be sure.

Look at the fans of cards and find the starting cards on the top of the fans. Although you can see the cards under the top card (because they are fanned), those cards cannot be used until they are on the top. It doesn’t matter which column you begin with and columns can “grow” at the rate the cards become available.

Here’s my game in progress.

I’m not showing you all 60 cards, but they’re all there for my game. As you can see, I have three out of the four columns started. I don’t have an 8 available yet to start the third column, but there’s a couple 8-cards I can see just below the top cards…..

With the cards I have showing here, there’s only move I can make. Can you see it? Let me talk out loud and share my thought process….

In the first column, 3 + 5 is 8. No eights available, although I do see two in the next layer down. One is under the 5 on the bottom left fan and the other is under the 9 up and to the left. I’ll see if I can use up that 5 or that 9. OK. Let’s look at the second column. 4 needs what to make 5? One. If I’m unsure, I can verify with the written starter numbers. Yup. It’s a 1. Do I have a 1? Oh yeah! Grab that and put in down. Now 4 + 1 is 5. I have three choices for a 5-card. Since I was looking to release the 8, I’ll take the 5-card from the bottom right fan.…

See how strategy makes a difference in this game? If you get to a spot where nothing can be played, pick up the fanned cards, shuffle, and lay the cards out in fans of three and see if you can win the game!

Here’s Clara playing the game.

Happy playing this week….. As always, drop us a comment and let us know how it’s going!